Teaching Estimating Radicals with Discovery

to Read (

Words)

Teaching estimating radicals using discovery

The first time I taught radical estimation in my 8^th grade classes I used organized steps and number patterns to introduce the lesson. I modeled, answered questions, and allowed for individual practice time. However, my students looked at me like I had two heads. I went home and thought carefully about how to make the lesson more concrete. Thus the Making Sense of the Irrational discovery worksheet was born (out of necessity for my kiddos). Describing distance between numbers using pictorial representations of fractions made a huge difference for my visual learners. Now I introduce my lesson using a discovery approach.

Provide Context

First, demonstrate why we need to estimate these radicals. Have students plot rational numbers on a number line. Note that it’s possible to be exact or very accurate. Then ask students to plot a few irrational numbers on a number line. This can open up a good discussion about how these numbers are estimated when placed on the number line because they are non-repeating, non-terminating decimals. This demonstration creates a good segway; although we do not have exact decimals for these numbers, we can estimate the value to the nearest tenth to give us an idea of the value of the number.

Introduce with Visuals

Now that the background knowledge has been established, use concrete models to help students visualize the distance between whole numbers.

Here’s how it works:

1) Identify the two perfect squares the radicand is between.

2) Take the square root of each of the perfect squares.

(The estimation is between these.)

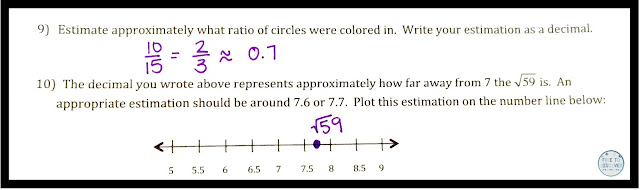
3) Find the distance between the two perfect squares. Draw this many open circles.

4) Find the distance between the smaller perfect square and the radicand. Color in this many circles.

5) The pictorial representation can facilitate a good guestimate for the decimal.

Draw Conclusions & Practice

At this point in the lesson, some students will continue with the concrete models and some will express “aha” moments and find shortcuts on their own. Making the lesson concrete first can help set a strong foundation for shorter, more efficient methods that students will more deeply understand. Consider allowing students to Think-Pair-Share about the methods they can use. Now they are ready to practice, practice, practice to make the skill permanent.

4 More Fun & Effective Practice Activities

Scavenger Hunt – Students move around the room as they practice estimating radicals. Each answer leads them to another station. A teacher and student favorite!!

Hands-On Number Line Activity – This hands-on activity allows students to work in groups to order real numbers. This activity can facilitate great discussions – especially because many of the numbers are close together.

Traditional Notes & Practice – This resource could make a great activity for day two to reinforce the procedures previously discovered. The best part is that it is differentiated based on readiness!