My students arrive to eighth grade
with a fairly strong knowledge of one- and two-step equations. This year with the new seventh grade
curriculum my standard math students are coming to me having never solved an
equation with the variable on each side. Within a couple of short weeks, we will review
two-step equations, discuss equations that involve the Distributive Property
and Combining Like Terms, solve many equations that have the variable on each
side; including those with fractional coefficients and special solutions, and
study word problems with scenarios modeled by linear equations.
Solving linear equations is an
immensely important skill for middle and high school students to master. It is imperative that students understand what
they need to do and why they need to do it. In this post, I would like to share some of
the strategies and resources I use in my eighth grade math classes.
1
– Socks and Shoes: Understanding “Reverse
Order of Operations”
Whenever possible, I try to relate
what we are doing in class to something students understand in their own
lives. Last week I compared equation
solving to putting on socks and shoes.
In the morning when getting ready for the day, socks go on first, then
shoes. But at the end of the day, shoes
come off first, then socks. The same is
true for equations. Consider the
equation 3x+1=-14. When the x “got
ready” using order of operations, it would have been multiplied by 3, then the
1 would have been added. So to undo the
operations, start by removing the 1 and then the 3. I have used this analogy year after year and
it has proven an effective strategy for my students.
2 – Require Beautiful Work:
Ban the “Baby Symbols”
I require very clear
equation-balancing evidence – even for one-step equations. I want students to understand why they can
add/subtract/multiply/divide. Very
quickly we move from one-step review to solving equations with four or more
steps. I have found that students who
are lazy about showing their work in the beginning of the unit tend to flounder
when they have many more steps, variables, and numbers to keep track of. I have also seen many more errors with
integers and incorrect operations when the work is not clearly done out. There are always some moans and groans, but I
know that it makes a huge difference a week later.
When students are showing their
beautiful work, I ban some of the symbols they may have used in the past. I “joke” that we are “breaking up” with them
and that it won’t be their last break up.
Eighth graders find it shocking that I bring in the idea of
relationships and it captures their attention.
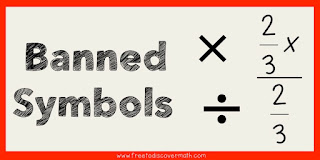
I do not allow students to use “x” for multiplication when solving. It can get confused with the common variable,
and parenthesis are a better way to communicate multiplication. I do not allow the traditional “divided by”
symbol either. I urge my students to use
the fraction bar to show their division.
I had a student tell me last week that he didn’t like the fraction bar
because then he couldn’t tell whether it was a fraction or division. Ahh! I
gave a couple of basic examples to show that they represent the same
thing. Finally, I do not allow students
to divide by a fraction. I require
multiplication by the reciprocal. I help
students buy-in by demonstrating how it actually saves them time and work if
they multiply directly within their organized steps.
3 – Use a Discovery-Based
Approach to Special Cases and Strategies
I am a big fan of balancing
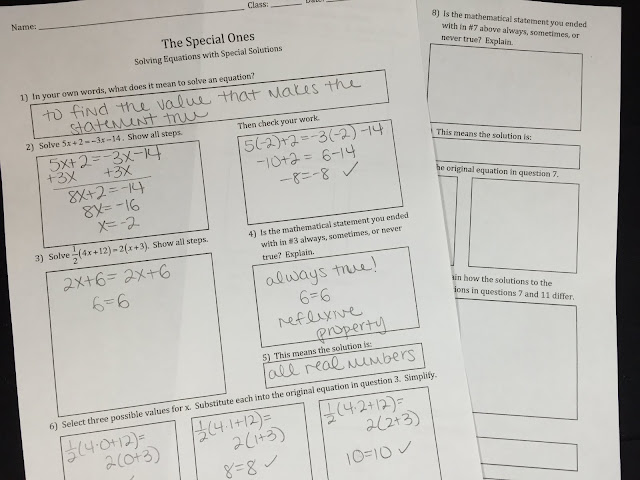
discovery-based learning and traditional practice. Special cases can be challenging to
understand. I group “x=0” in with
special cases because it often is confused with no solution. To help students build meaning and understand
no solution and all real numbers, I created discovery-based worksheets that
lead students to the big ideas. They
learn, not just what to look for in order to identify the special solutions,
but what it actually means for there to be a special solution to an equation.
I use a similar approach when
teaching students about equations that involve fractions and decimals. I created a discovery-based worksheet that
walks students through how to clear out fractions and decimals from
equations. They learn how to select the factor
they should multiply by on each side of the equation, and they clearly observe
how much easier an equation can be to work with when taking the time to clear
out the fractions or decimals from the start.
4 – Practice in a Fun and
Engaging Way
The best way for students to master
linear equation solving is to do lots of practice. But the practice does not need to be
worksheet-based. Here are some of the
activities I use to help students learn to solve equations:
Holiday-Themed
Partner Practice: My first few years of teaching, holidays would roll around
and I would get so excited! I wanted to
acknowledge the holiday in my classroom, but I could never find anything
mathematically meaningful. That’s why I
decided to create my line of holiday-themed partner stations! Students travel in pairs as they each solve a
different problem. If they are correct,
they will have the same answer as their partner. Great self-checking activity. Fun unscrambling component at the end, too!
·
Front Whiteboard Practice: My eighth graders love to write on my whiteboard. I call five students up to board at a time. Everyone else has paper and pencil out so they continue practicing even if the spotlight is not on them. I read an equation aloud that everyone solves at the same time. I switch up which students are at the front board so that everyone goes up 2-3 times.
Front Whiteboard Practice: My eighth graders love to write on my whiteboard. I call five students up to board at a time. Everyone else has paper and pencil out so they continue practicing even if the spotlight is not on them. I read an equation aloud that everyone solves at the same time. I switch up which students are at the front board so that everyone goes up 2-3 times.
Mini-Whiteboard
Practice: I have a set of mini-whiteboards to use with my classes. Students solve an equation on the board, then
when I give the signal they all hold up the boards with their work and the
answer so I get quick feedback on the level of understanding in the room and I
can pinpoint students that I should check in with.
Task Cards: I love task cards! My favorite strategy for task cards has been to leave them in a bin in the front of the room with the answer key posted nearby. Each student takes one card, checks their answer, and replaces their card with a new one once they get it correct. I have done this individually or in pairs.
Scavenger Hunt: I purchased a CSI-type scavenger hunt to use with my students. I hid the
“clues” around our Auditorium and we took a “field trip.” Students loved getting out of the regular
classroom setting, working at their own pace and in an unconventional way, and
filling in the answers to the mystery during the process. Thank you 21st Century Math Projects!
This post is also featured on the TpT blog!
Subscribe to the Free to Discover blog here:
No comments:
Post a Comment